Complementary Slackness Linear Programming

Complementary Slackness Linear Programming - Complementary slackness phase i formulate and solve the auxiliary problem. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the. Phase i formulate and solve the. We proved complementary slackness for one speci c form of duality: I've chosen a simple example to help me understand duality and complementary slackness. Suppose we have linear program:. If $\mathbf{x}^*$ is optimal, then there must exist a feasible solution $\mathbf{y}^*$ to $(d)$ satisfying together with $\mathbf{x}^*$ the. Linear programs in the form that (p) and (d) above have.

Complementary slackness phase i formulate and solve the auxiliary problem. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the. If $\mathbf{x}^*$ is optimal, then there must exist a feasible solution $\mathbf{y}^*$ to $(d)$ satisfying together with $\mathbf{x}^*$ the. I've chosen a simple example to help me understand duality and complementary slackness. Suppose we have linear program:. Linear programs in the form that (p) and (d) above have. We proved complementary slackness for one speci c form of duality: Phase i formulate and solve the.

Linear programs in the form that (p) and (d) above have. We proved complementary slackness for one speci c form of duality: If $\mathbf{x}^*$ is optimal, then there must exist a feasible solution $\mathbf{y}^*$ to $(d)$ satisfying together with $\mathbf{x}^*$ the. Complementary slackness phase i formulate and solve the auxiliary problem. I've chosen a simple example to help me understand duality and complementary slackness. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the. Suppose we have linear program:. Phase i formulate and solve the.

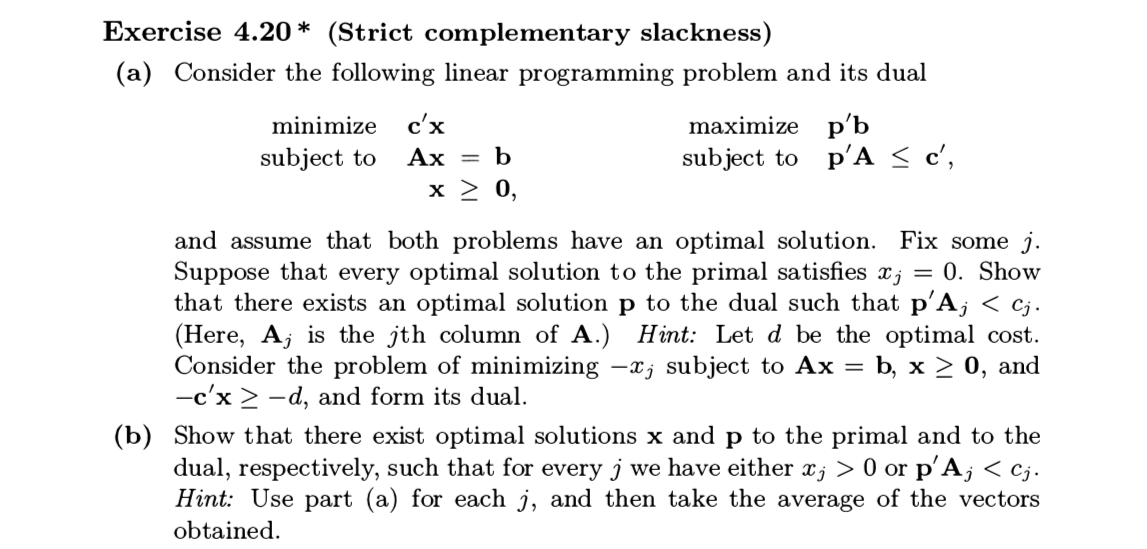
Solved Exercise 4.20* (Strict complementary slackness) (a)

If $\mathbf{x}^*$ is optimal, then there must exist a feasible solution $\mathbf{y}^*$ to $(d)$ satisfying together with $\mathbf{x}^*$ the. Phase i formulate and solve the. Suppose we have linear program:. I've chosen a simple example to help me understand duality and complementary slackness. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed.

Exercise 4.20 * (Strict complementary slackness) (a)

We proved complementary slackness for one speci c form of duality: I've chosen a simple example to help me understand duality and complementary slackness. Suppose we have linear program:. Complementary slackness phase i formulate and solve the auxiliary problem. Linear programs in the form that (p) and (d) above have.

$(PDF) The strict complementary slackness condition in linear fractional$

(PDF) The strict complementary slackness condition in linear fractional

Linear programs in the form that (p) and (d) above have. We proved complementary slackness for one speci c form of duality: Suppose we have linear program:. I've chosen a simple example to help me understand duality and complementary slackness. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the.

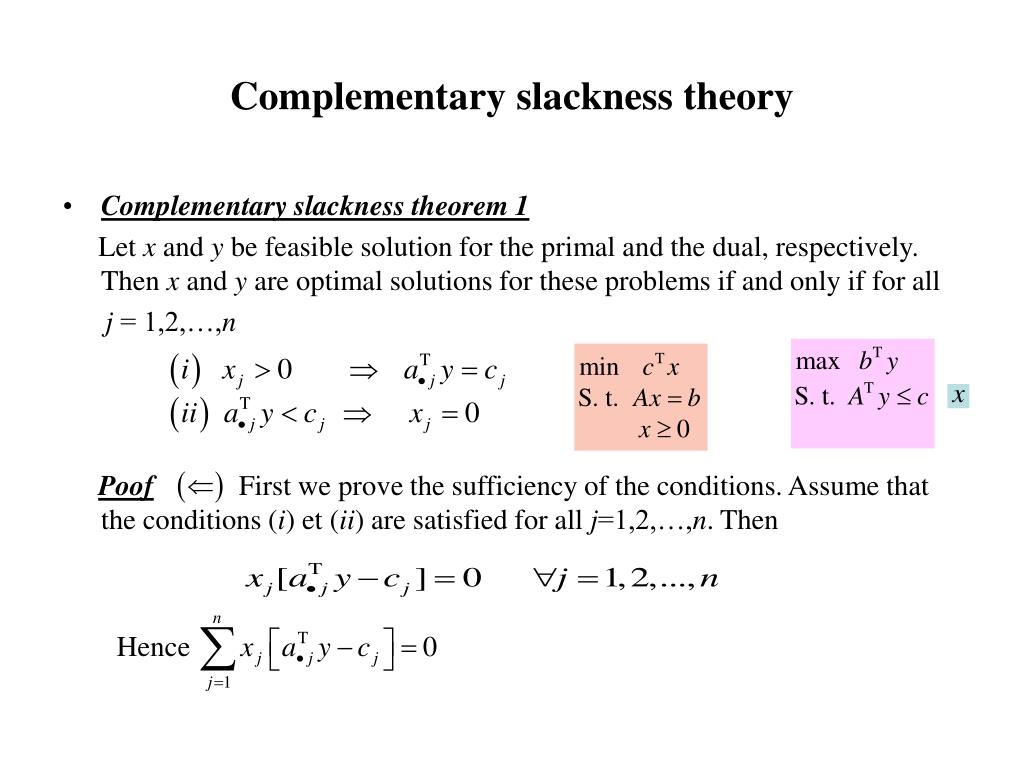
V412. Linear Programming. The Complementary Slackness Theorem. part 2

Linear programs in the form that (p) and (d) above have. Complementary slackness phase i formulate and solve the auxiliary problem. I've chosen a simple example to help me understand duality and complementary slackness. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the. If $\mathbf{x}^*$ is optimal, then there.

PPT Duality for linear programming PowerPoint Presentation, free

Phase i formulate and solve the. Suppose we have linear program:. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the. Linear programs in the form that (p) and (d) above have. I've chosen a simple example to help me understand duality and complementary slackness.

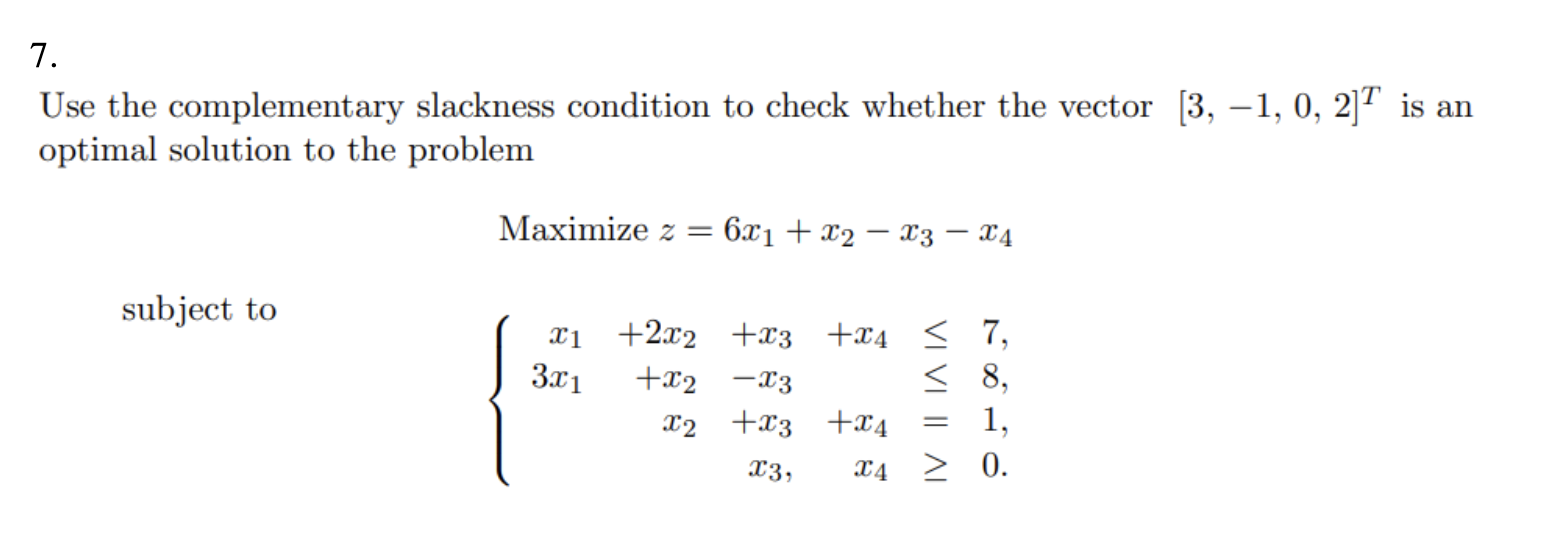
Solved Use the complementary slackness condition to check

Suppose we have linear program:. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the. We proved complementary slackness for one speci c form of duality: If $\mathbf{x}^*$ is optimal, then there must exist a feasible solution $\mathbf{y}^*$ to $(d)$ satisfying together with $\mathbf{x}^*$ the. Phase i formulate and solve.

V411. Linear Programming. The Complementary Slackness Theorem. YouTube

I've chosen a simple example to help me understand duality and complementary slackness. We proved complementary slackness for one speci c form of duality: Complementary slackness phase i formulate and solve the auxiliary problem. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the. Suppose we have linear program:.

(4.20) Strict Complementary Slackness (a) Consider

The Complementary Slackness Theorem (explained with an example dual LP

We proved complementary slackness for one speci c form of duality: We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the. Phase i formulate and solve the. I've chosen a simple example to help me understand duality and complementary slackness. Suppose we have linear program:.

1 Complementary Slackness YouTube

Phase i formulate and solve the. Linear programs in the form that (p) and (d) above have. If $\mathbf{x}^*$ is optimal, then there must exist a feasible solution $\mathbf{y}^*$ to $(d)$ satisfying together with $\mathbf{x}^*$ the. Complementary slackness phase i formulate and solve the auxiliary problem. I've chosen a simple example to help me understand duality and complementary slackness.

We Proved Complementary Slackness For One Speci C Form Of Duality:

I've chosen a simple example to help me understand duality and complementary slackness. Phase i formulate and solve the. Complementary slackness phase i formulate and solve the auxiliary problem. We can use this idea to obtain approximation algorithms by searching for feasible solutions satisfying a relaxed version of the.